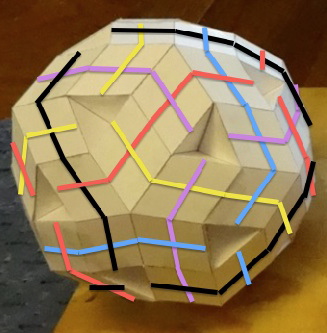
Quintetra Blocks
|
photos by Frans de Vreugd
|
These assemblies are made up of 30 unusually shaped blocks that easily go together to surround a hollow space.
On the outside of the assembly there are five sets of four indented regular tetrahedral spaces, hence the name Quintetra. In each set, the four tetrahedrons are positioned so that the points closest to the center are the four vertices of a larger regular tetrahedron. Inside of the triacontahedron box that fits snugly in the middle of the arrangement, there's yet another magnetic assembly (see video below) that surrounds a rhombic dodecahedron. The angle in each of the 60 external congruent rhombuses is approximately 113.86º. We have found very many more interesting 3-dimensional shapes that can be made from equilateral triangles and 113.86º parallelograms. To make them yourself out of paper, feel free to download this pdf file that we presented at the Gathering 4 Gardner conference in Atlanta in 2014:
|
Click on the images to enlarge.
Tigerwood, Maple, Bubinga, Burmese Blackwood
(4" diameter)
Photography by Dean Powell
|
Making the Quintetra Blocks:
|
